Weaving our knowledge together: Uncovering Pasifika learners’ mathematical funds of knowledge

Introduction and background

Both in New Zealand and internationally, diverse groups of people including Indigenous, migrant, and other minority communities are underrepresented in mathematics with continuing inequitable outcomes from education. Many studies (e.g., Bartell et al., 2017; Joves et al., 2015) recognise the barriers for diverse learners in accessing education when culturally embedded ways of knowing, or cultural capital, are not aligned with what is privileged in schooling practices. Researchers argue that equity in schooling can only be achieved when educators explicitly connect to and build on the cultural, social, and linguistic contexts of nondominant students (Bartell et al., 2017). This includes developing understanding of the identities of learners and the “funds of knowledge” they acquire in their lives outside of school (Joves et al., 2015; Moll et al., 1992; Parker et al., 2017). Aligned with a funds of knowledge approach, a key policy shift within New Zealand has focused on schools “localising” the national curriculum to align with the context and aspirations of the local community and student population (Trinick & Heaton, 2020). However, developing a meaningful, aligned local curriculum requires teachers to understand and value the connections between students’ home and community activities across all areas of the curriculum including mathematics (Aguirre et al., 2013; Llopart & Esteban-Guitart, 2017; Rubel & McCloskey, 2021).

The project “Weaving our knowledge together” focused on recognising and recording the mathematical funds of knowledge of young Pacific students, sharing this with teachers, and then using the data to develop and enact culturally relevant, mathematically challenging, group-worthy tasks. Over a period of 3 years, the project involved 42 families and their children from two primary schools and 20 teachers. Drawing on a two-phase cyclical approach, the research team first worked with the young Pacific learners and their families to support them to document their mathematical funds of knowledge and share stories of their daily lives at home and in the community that involved mathematics. The second phase of each cycle involved collaborative work with the teams of teachers to share the data related to students’ mathematical funds of knowledge and draw on this in task design followed by trial task enactments.

This report begins with an overview of literature related to mathematical funds of knowledge and developing and enacting group-worthy contextual tasks. The research questions are provided, followed by the research methods, key findings, implications for practice, and conclusions from the study. All aspects of the study were impacted by the COVID-19 pandemic due to the lock-down and school closures. This resulted in the need to cancel and postpone interviews, meetings, and observations, change the numbers of participants, and extend the data collection period.

Mathematical funds of knowledge

The funds of knowledge theoretical framing recognises that all people and cultures have bodies of knowledge and skills that are historically accumulated, culturally developed, and support individual/ household functioning and wellbeing (Moll et al., 1992). However, in New Zealand and internationally, there have been few research studies that focus on the way families from marginalised communities interact in ways related to mathematics outside of school settings (Civil, 2016; Mills et al., 2019; Williams et al., 2020). International studies exploring the mathematics of marginalised communities have generally involved interviews with parents and caregivers to document the mathematical activity present in household routines (Takeuchi, 2018; Williams et al., 2020). These studies illustrated that parents often have initial difficulties identifying mathematics in household activities beyond cooking and construction. Other studies (e.g., Mills et al., 2019; Zipin et al., 2012) have broadened the traditional data collection approaches used in funds of knowledge research by positioning children themselves to document their funds of knowledge. These researchers have noted that this enables the collection of a wide range of experiences including both unconventional and implicit scientific knowledge. Our project “Weaving our knowledge together” built on these previous studies with a focus on recognising and recording the mathematical funds of knowledge of Pacific learners in Aotearoa/New Zealand.

Developing and enacting group-worthy contextual tasks

Group-worthy tasks provide opportunities for students to engage in collaborative activities that build connections between mathematical ideas for themselves, require struggle and persistence, and demand high-level cognitive processing (Smith & Stein, 2011). These task types build on the following characteristics: illustrate important mathematical concepts; include multiple steps and allow for multiple representations; have several possible solution paths, are mathematically challenging; and activate educationally powerful connections. In this way, group-worthy tasks and associated learning activity offer multiple ways to support powerful learning for diverse learners. They provide all students with access to challenging, worthwhile mathematics and opportunities to engage in a range of mathematical practices: questioning and reasoning; connecting ideas; making mathematical generalisations; and using representations (National Council of Teachers of Mathematics, 2014). Positioning students as doers and sense makers of mathematics affords a redistribution of the mathematical authority in the classroom (Langer-Osuna, 2016).

Contextual tasks can take the form of traditional story problems to open-ended modelling tasks and build on both realistic and imaginary contexts (Wernet, 2017). These tasks can be motivating and provide an entry point to deepen reasoning and allow students to connect everyday knowledge with the different solutions (Betts & Rosenberg, 2016; Clarke & Roche, 2017; Wernet, 2017). While the use of contextual tasks has the potential to engage and support students to make connections between their home and school use of mathematics, some researchers (e.g., Clarke & Roche, 2017; Wernet, 2017) highlight that careful consideration needs to be given to both the choice of context and task implementation. Importantly, Averill and Clark (2012) and Wernet (2017) both caution that it can be difficult to design tasks that align with student interests and experiences and that quality group-worthy tasks drawing on cultural contexts are difficult to design.

Overall aims and research questions

The overall aims of this project were threefold: to (i) document the multiple mathematical funds of knowledge of young Pacific people and their families by developing practical strategies to empower the participants to recognise their mathematical funds of knowledge and share these with educators; (ii) support the equity agenda in schooling by examining how group-worthy mathematical tasks drawing on funds of knowledge are developed by teachers; and (iii) investigate the influence of culturally located tasks on students’ mathematical learning, engagement, and disposition. In relation to the aims, the following research questions were investigated:

1. What are the different mathematical funds of knowledge of young Pacific people and their families in New Zealand?
2. How can we as educators (both researchers and teachers) better understand and value the mathematical connections within diverse students’ home and community activities and artefacts?
3. How can the application of students’ experiences and their funds of knowledge be incorporated within group-worthy mathematical tasks?
4. How can funds of knowledge models be used to enhance equitable learning opportunities within the mathematics classroom?
5. How do opportunities to learn through group-worthy tasks based on a cultural context affect students’ development of conceptual understanding of mathematics?
6. How does engagement with group-worthy tasks based on cultural contexts influence students’ perceptions of mathematics and their role as mathematics learners?

Research design

The study used a methodology of participatory design research (Bang & Vossoughi, 2016; Cumbo & Selwyn, 2022; Kelly et al., 2014; Liebenberg, 2018; Sutton-Brown, 2014). We built on the underlying principles of inclusion and partnership where the research team and participants were involved in the data collection and worked together to advance social justice and equity agendas. Drawing on the framing of funds of knowledge, we took an inclusive view of education that included families/communities, students, and teachers and began from the premise that students enter schooling with funds of knowledge, and that teachers need to become learners of their students’ multiple knowledge bases (Turner et al., 2012). This meant that the learning setting in the project was expanded to include both inside and outside of school contexts. Our focus in the first phase of each cycle was empowering young Pacific people and their families to recognise and document their mathematical funds of knowledge so that this could be shared with the research team including the teachers. The design research approach was used to address at both a pedagogical and theoretical level the results of the active innovation and intervention in the mathematics classrooms (Kelly et al., 2014). The focus in the second phase was on task design drawing on the funds of knowledge and culturally located contexts and trial task enactment. Throughout the project, a range of data collection tools and analysis methods were used which will be described in a section below.

Partners and settings

Two primary schools in Auckland were invited to participate in the study. AME Primary School had three teachers participate across a 2-year period. This included one teacher of Pacific heritage, European heritage, and South Asian heritage. AMB School had eight teachers participate in the first year including two teachers of Pacific heritage, one teacher of South Asian heritage, one teacher of East Asian heritage, and four teachers of European heritage. In the second year of the project, 18 teachers at AMB School participated in the project, including four teachers of Pacific heritage, four teachers of Māori heritage, two teachers of South Asian heritage, two teachers of East Asian heritage, and six teachers of European heritage. Both sites had significant disruption due to the COVID-19 pandemic and school closures during the data collection period. In total, 42 families and their children (aged between 5 years and 11 years) participated in the project. These families were of differing ethnicities including Samoan, Tongan, Niuean, Cook Island Māori, Fijian, New Zealand Māori, and African but were predominantly of Pacific heritage with many of multiple ethnicities. Of the 42 families, two families had Samoan as their first language and two families had Tongan as their first language; the remaining 38 families spoke English as their first language.

Data collection methods and data analysis

Forms of data to address three key areas and focuses were collected over the period of the study:

Documentation of Pacific learners’ funds of knowledge

1. Two semistructured interviews were undertaken with family participants (parents and caregivers) at the beginning and end of the project. The initial interview examined their family history related to their educational experiences and job history as well as perspectives of current everyday practices and artefacts related to mathematics. The final interview focused on perspectives of home, community, and cultural activities that related to mathematics and the shifts in perception and learning related to mathematics in the home and community from being involved in the project. These were conducted in the language selected by the family participants and in two cases the interviews were conducted in Tongan and Samoan.
2. Visual data were collected in the form of photographs taken by student/family participants to capture activities and artefacts that connected to mathematics. Photo-elicitation interviews using a basis of storytelling were undertaken with the students on a 6-weekly basis throughout the project. Students were asked to describe what was happening in the photo and why they took it.
3. Workshops with family participants were video recorded. During the workshops, the photographs and stories shared by the students were shared with the family participants. Collective analysis of the images and photographs was undertaken with a focus on the mathematical strands evident in the activity or artefact and of the types of activity or artefact.

Documentation of educators’ learning and use of mathematical funds of knowledge in lessons

4. Two semistructured interviews were used at the beginning and end of the project to map the knowledge and understanding of teachers in relation to student and family mathematical funds of knowledge. The final interview focused on the changes in planning and teaching mathematics as well as growth in understanding funds of knowledge of Pacific students and their families.
5. Video-recorded collaborative planning workshops were used throughout the project. In the first instance, slideshows of the photographs and stories from the students were shared with the teachers. The teachers and research team engaged in discussion about the strands of mathematics evident in the activities and artefacts. Collaborative activity was undertaken to plan a series of mathematical tasks aligned with a strand of mathematics and drawing on the contexts and funds of knowledge of the students. Planning included developing the task, mapping out the mathematical language and Pacific/Māori language resources of the students, planning how to launch the task in a way that connected and honoured student knowledge and funds of knowledge, and anticipating possible student responses to the task.
6. Video-recorded task enactments during lessons. Within each lesson, the video records targeted specific lesson events including the launch where the teacher facilitated the students to connect to the context of the tasks, teacher interaction with students working in small groups, and the concluding large-group discussion and plenary. The videos provided a rich data set to focus on growth in teacher expertise in relation to the enactment of culturally located, group-worthy mathematical tasks.
7. Written or audio-recorded individual or group teacher reflections on the lessons. This included reflection on the launch, teacher actions throughout the lesson that facilitated students to connect with multiple knowledge bases including funds of knowledge, mathematical knowledge, language resources and values, and reflection on the mathematical learning of students.

Data collection of student outcomes related to the tasks including participation, engagement, and conceptual mathematical development

8. Two semi-structured interviews were used at the beginning and end of the project. Student participants were asked about current learning experiences related to mathematics and activities in home and community settings that connected to mathematics.
9. Video-recorded task enactments during lessons were used to focus on student participation in mathematics lessons and their responses to the mathematical tasks. This included video analysis of students’ collective development of mathematical thinking and learning both in small-group work and during large-group discussion.
10. Audio-recorded individual post-lesson reflections. Students were asked to reflect on the lesson including their mathematical learning, connections to the task context, and their enjoyment and engagement in the lesson.

To ensure that the design of the study was informed by evidence from the field, including the research team and teachers’ developing understanding of the mathematical funds of knowledge of Pacific communities and how to enact the tasks most effectively in the classroom, data collection and data analysis occurred simultaneously throughout the project. The research team engaged in a process of continuous reflection to adjust and modify the collaborative planning workshops and to support the teachers in the enactment of the tasks in the classroom.

Retrospective data analysis used a mixed inductive and deductive approach along with a set of guiding questions related to the research focus. For each set of data, multiple levels of coding were developed and used.

To investigate the first set of data related to mathematical funds of knowledge of Pacific communities, we first drew on previous work related to funds of knowledge by Moje et al. (2004). Initially, the data were coded using the umbrella codes developed by Moje et al. (2004):

1. family
2. community
3. peer
4. popular culture.

Following the initial coding, we also included an umbrella code based on Esteban-Guitart’s (2012) development of funds of identity, an extension of funds of knowledge. Sub-codes were developed under the umbrella code; for example, a description of playing with playdough with friends was coded under peers and the sub-code, play. Data analysis and coding was then repeated at a second level to investigate the different strands of mathematics apparent in the photograph and storytelling data. There were six umbrella codes used for this coding: algebra/patterns; measurement; geometry; statistics; probability; and number.

The second set of data focused on educators’ learning and use of mathematical funds of knowledge in lessons. The third set of data related to student outcomes regarding the mathematical task enactment with a specific focus on participation, engagement, and developing conceptual mathematical understanding. Both these sets of data were analysed in a similar way or in parallel. The teacher and student interview responses were analysed by developing themes and patterns for each question and then looking for patterns across the questions. Document analysis was undertaken from the task planning sessions to examine the tasks that were developed including links to the photographs and student interview data and teacher anticipations.

To analyse the video-recorded lesson observations, we began by dividing each video recorded lesson into three event segments:

1. launch of the task
2. small-group work
3. large-group discussion.

The event segments were then analysed using four umbrella codes that focused on connections that the teachers made to the multiple knowledge bases of students with a fifth code focused on missed opportunities. The codes related to connections included the:

1. contextual features of the task
2. cultural background and heritage of the students
3. conceptual mathematical knowledge
4. mathematical practices.

The second level of coding examined the teacher pedagogical actions and related student responses that were represented under each of the connections as well as moments during the lesson that represented missed opportunities. Both the teacher and student reflections on lessons were analysed thematically and used to validate and verify patterns and themes noted by the research team.

Ethical processes

A research ethics approval was provided from the Massey University Human Ethics review panel.

Consideration was given to the need for informed consent when undertaking research with children. In this study, children were asked to give fully informed consent in collaboration with the approval of those who were their guardians. Both verbal and written information was provided to facilitate this process. The written information and consent forms were translated into appropriate Pacific languages as needed and modified for the children to understand. To maintain informed consent throughout, the children were asked for verbal consent prior to any interviews and advised that they could choose whether to answer the questions and ask for the recording device to be turned off at any time. At all times, the research was conducted in accordance with the Massey University Code of Ethical Conduct for Research, Teaching, and Evaluations involving Human Participants.

Key findings

In this section, we present a selection of key findings from the overall research project related to the three aims of the study. We begin by presenting the key findings related to the first aim of the study: to document the multiple mathematical funds of knowledge of young Pacific people and their families by developing practical strategies to empower the participants to recognise their mathematical funds of knowledge and share these with educators.

Key finding 1:
Engaging in documenting mathematical funds of knowledge with the use of photography and photo-elicitation provides a means for young Pacific people and their families to recognise and share their mathematical funds of knowledge.

Initially, at the beginning of the project, many of the participants (parents and children) had difficulty describing where mathematics could be seen in their home and community environment and activities beyond school-like contexts. Typically, they described either homework activities or those related to number identification or counting. For example, one family described recently purchasing a poster of multiplication facts to display in their lounge to help their children learn these and make it normal for when they would see it in the classroom. Other families described counting shells that they had collected at the beach or counting out the number of plates and utensils when setting the table. Beyond these types of school mathematics contexts, mathematical funds of knowledge related to measurement in cooking were identified and described: “How many cups of water to put in the pot to boil.” Interestingly, parents were able to identify a range of ways in which they used mathematics in their employment and job roles.

Aligned with the initial interviews, in the early photographs and photo-elicitation interviews, we noticed a heavy focus on numbers, digits, and counting. This included photographs of mathematics homework and basic facts practice, as well as digits that participants photographed in the community or descriptions of counting sets (see Figure 1).

FIGURE 1. Initial photographs from participants focused on numerical situations

“These are my two and three times-tables. I just learnt them this year. I did it by myself.” Year 4 student	“We see signs and stuff. Two and five make 25.” Year 2 student

It appeared that, at the beginning of the data collection period, many of the participants had a narrow view of mathematics that was influenced by a focus on number and counting. Despite the predominance of documenting numbers or viewing activities through a numerical counting lens, interestingly, we noted several of the younger participants in the early years of schooling took photographs of play activities and artefacts that drew on richer threads of mathematics including measurement and geometry (see Figure 2). To ensure that participants drew on a lens of mathematics beyond number, we undertook sharing and discussion sessions with the child participants during which we shared a range of different photographs and the related stories with others. These activities were also undertaken with the adult participants in the study when the COVID restrictions allowed.

FIGURE 2. Initial photographs from younger participants involving play with links to measurement and geometry

“This is slime, I had one and my friend had one and we mixed it together and it made that colour. You need glue, borax, and food colouring, and you can use shaving crème if you want fluffy slime This isn’t fluffy slime, it’s normal slime. Sometimes we add glitter. We were pretending to bake so we made small cookies, and we rolled them into balls and then squashed them down and they spread out like cookies.” Year 3 student

“I took a photo of this because it’s big. It’s like paper so you can wrap people’s presents. I like to wrap my toys and see if it fits.” Year 1 student

Importantly, we note that the questions used during the photo-elicitation interviews focused on the participants telling the story of the activity or artefact rather than describing the mathematics that they saw. We argue that this was important in developing a rich background to the photographs which the research team and teachers could then view through a broad mathematical lens. As the project progressed, we saw participants begin to make connections to different strands of mathematics across a range of activities and artefacts as highlighted in the next section. It was also noticeable that some of the participants began to mathematise everyday situations and ask mathematical questions about these (see Figure 3).

FIGURE 3. Photographs and interview data mathematising everyday situations

“I was wondering how much motor you need to make 100 miles per hour for the bigger boat.” Year 6 student	“We were looking at how much bark you would need to cover the bottom of the playground. Because when you go on the monkey bars and you jump off, if you go on the ground then you can slip and fall and bang your head.” Year 5 student

Using photography appeared to be a fruitful way of positioning young Pacific learners and their families to recognise and share their mathematical funds of knowledge. Both the students and their parents alike commented on this in the final interview at the end of the project. For example, one student reflected: “There is maths literally everywhere. There is basically no place I can look that there is not maths.” Similarly, in the final interviews, parent participants commented on both their shift in perception of mathematics and a deepening insight into different elements of mathematics.

When we first started taking photos and that’s when I kind of was like ‘Oh my God it’s [maths] actually like everywhere’. I didn’t really pay attention before but now; it’s to me like since taking those photos that like you can see [maths] everywhere.

There really is a misconception that maths is just numbers and really, it’s not. There are other strands to it, there’s a bigger picture. The world revolves around maths, its everywhere.

I have actually learned that maths is everywhere and you don’t really think about it, but you realize that maths is literally all around you at all times, whether it be in nature or whether it be in your house and you’re looking at shapes, or you’re looking at patterns, or you’re dealing with money, or whatever it might be, it just really truly is all around you. So that’s probably something I think I’ve learned, maths is in everything that we do and everything that we see.

Overall, taking photographs and sharing these with the research team positioned young Pacific learners and their families as experts of the mathematics present in their own lives. The photographs both provided a context to talk about life outside of school and also a tool to recognise mathematics.

Key finding 2:
Young Pacific people and their families have a range of mathematical funds of knowledge connected both to cultural artefacts and participation in differing activities.

Young Pacific people photographed and shared a range of mathematical funds of knowledge that were connected to various settings and activities. More information can be found about this key finding in an article (Hunter, 2022; see Reference list for details). Analysis of the photographs and stories illustrated that, most commonly, these related to their family funds of knowledge—those activities that the children undertook with their family. Second most common, participants took photos that connected with their funds of identity. These were personal life experiences that students referred to in relation to their identity. The next most common context was photographs connected with community activities and these related to activities and experiences that the students undertook within their local community. Least common were photographs that were related to activities with peers and activities that related to popular culture. It appeared that, given the age of the participants in the study, many of their experiences were with their families. It may also be possible that the data collection in relation to community and peer activities was impacted by the COVID-19 pandemic and the restrictions on community activities and opportunities to socialise with peers.

Each set of activities, experiences, and artefacts related to students’ mathematical funds of knowledge underwent a second layer of analysis to specifically examine the context of the activity or artefact. Table 1 shows the contexts related to each fund of knowledge in order of the frequency with which they were coded. Across these activities and artefacts, participants both implicitly and explicitly described connections to mathematics.

TABLE 1. Context and sub-code of the context in order of frequency

Family	Identity	Community	Peer	Popular culture
Cooking/food	Toys/play	Social events	Sports	Video games
Cultural artefacts	Artefacts	COVID	Play	Online activity
Chores/household tasks	Arts	Community events	Trading cards
Games	Video games
Financial situations	Culture
Physical activity	School
Arts

Family funds of knowledge were most prevalent in the photographs and stories shared by the students. To illustrate and provide insight into the findings, some of the stories of mathematics related to family funds of knowledge gathered from the participants during photo-elicitation interviews will be shared along with the analysis of these. Most commonly, students shared photographs related to food and cooking with their families. These included pictures of cooking, baking, gathering food, and pre-packaged food. In some cases, students described growing food in the garden and gathering fruit such as mandarins from fruit trees. Other examples included students describing baking activities for cookies and cakes or making drinks such as otai or fruit smoothies (see Figure 4).

FIGURE 4. Family funds of knowledge related to preparing food

“I was about to make a smoothie and I noticed that it was maths because of measuring the ingredients. When I make mine, I usually measure most of my ingredients, because sometimes it could taste like too much yoghurt. Maybe it would be too watery if you added too much water without measuring. I thought that measurement in maths would be the litres. So first of all, you open the banana, put the banana in and then you’d add the mango, we use the pulpy version. Then you add 2 spoons of yoghurt, 1 cup of blueberries or any frozen berries and pineapple, and then you blend it in the blender for about a minute 30 seconds.” Year 6 student

In the example in Figure 4, we saw the participant recognising that preparing food involves developing measurement concepts both in relation to weight and capacity. It was also apparent that the participant was informally considering concepts related to proportion and ratio regarding both the taste and texture of the smoothie and measurement of ingredients. In other cases, we saw participants describing the fractional amounts of ingredients that would be used when cooking.

Participants also commonly shared photographs of traditional craftwork and cultural elements from their home environments such as tattoos and items of dress. This included sharing the cultural significance of the craftwork, describing how they were made, and how they would be worn (see Figure 5).

FIGURE 5. Family funds of knowledge related to cultural activities

“These are from Samoa and they are traditional bowls. Mostly they use the bowls for doing dances and they put in in front of you and one girl is sitting down and they scrub the bowl and they grab a coconut cup and they dip it into the coconut water and they give it to special people. I saw it live for my Dad, my Aunties were dancing for my Dad”. Year 6 student	“It is an ‘ei katu, it’s like a headpiece. It’s a Cook Island way of looking flawless and pretty. My Uncle makes these and he sells them to make money. He has to measure how wide the head is so he has to measure their head and then he makes it with a kind of Material.” Year 5 student
“This is Fijian masi. It is made from bark from the trees. We have it at home and it has lots of patterns on it.” Year 3 student

Other examples provided by the students explicitly identified aspects of geometry by describing the shapes and motifs used in carvings such as wooden axes or such as on the turtle (in Figure 5). In some cases, this included identifying geometric properties such as translation and enlargement. A connection to algebra was evident in the description of the repeating patterns on the Fijian masi. Additionally, in the second example in Figure 5, it was evident again that the participants recognised the use of measurement in making an ‘ei katu. Implicit in both of the first two photographs and descriptions were opportunities to connect to algebra and patterns through those used in dance and in the flowers on the ‘ei katu.

Also evident as a common source of mathematical funds of knowledge were the activities related to chores and household work that both young Pacific learners and their parents engaged in. Given that the data collection period included significant times when the country was in lock-down, some of the photographs and descriptions reflected this in referring to home improvements, while others were everyday household chores (see Figure 6).

FIGURE 6. Family funds of knowledge related to chores and household activities

“This was when we were tiling at Grandma’s house. We stayed there for lockdown with one of our parents. We filled it up to the wire part but we had to use those little crosses sticking out to make sure we keep it at that height and make sure it stays straight. Then when it dries, you can take them out. We helped our Uncle do it and then we used water and next morning. We got to sponge it down and then scrape it off.” Year 5 student

“We both have a turn on the vacuum and my Mum times us. Then it is my Mum’s turn and then when her time is up, it is my sisters’ turn. My turn is for about 30 minutes and my sister has 30 minutes. My Mum is about 50 minutes and she has to vacuum the rest of the whole house and all the rooms. We have to move everything around.” Year 5 student

Rich connections to mathematics were evident in the photographs and descriptions of household activities and chores. Both geometrical and measurement concepts are an important element of successfully tiling space as this involves length and area measurement as well as an understanding and application of tessellation. Similarly, in the description of undertaking chores such as vacuum cleaning, the student participants referred to the measurement of time and space within the household.

These examples illustrate the rich and varied contexts in which young Pacific learners and their families engage in activities aligned with mathematics and the ways in which mathematics is visible within everyday and cultural artefacts. Through the photographs and photo-elicitation interviews, we have been able to share a view of the mathematical funds of knowledge that are not typically recognised or presented within mathematics classrooms.

Key finding 3:
It is important for teachers to have opportunities to learn about diverse students’ funds of knowledge related to mathematics. Drawing on student experiences and their funds of knowledge to develop group-worthy mathematical tasks requires careful thought and detailed planning.

Further information related to this key finding is described in an article (Hunter et al., 2020; see Reference list for details). At the beginning of the study, most of the teacher participants identified an important factor in designing mathematical tasks as the need to consider home culture, experiences, and background. However, it was challenging for teachers to both identify funds of knowledge related to mathematics and to use these to develop group-worthy mathematical tasks. Throughout the study, to support the teacher participants to learn about their Pacific students’ lives and experiences outside of school, we engaged in termly planning meetings. Each of the meetings began with a slideshow of photographs that the students had taken along with the stories provided from the photo-elicitation interviews. As one of the teachers reflected mid-way through the project, this highlighted to her that “children have a unique perspective on what maths is and how they perceive it in their everyday lives”. From this teacher’s perspective, centring the task development in the experiences of the students meant that the students “have more success because they understand the context and what should be happening”.

Key to the task development was both understanding the contextual information and looking for authentic connections to mathematical strands represented in The New Zealand Curriculum. The planning process included looking at all the photographs and stories and noting down specific connections to the mathematical strands that would be taught in the upcoming terms. This meant that, at times, the same example was used to teach different strands of mathematics. The teachers then worked with the research team to map the concepts and big ideas related to the mathematical strand to be taught before planning mathematical tasks that were connected to the photo context shared by the students. These became part of a larger 5-week mathematics unit and teaching plan. As part of the planning process, the teachers and researchers collaboratively discussed how the tasks could be launched in a way to connect to the context and anticipated potential student responses to the group-worthy tasks.

Table 2 shows examples of the photographs and photo contexts related to the funds of knowledge shared during photo-elicitation interviews and the mathematical tasks that were collaboratively developed by the teachers and research team from these data.

TABLE 2. Image, context, and mathematical task

Photo	Photo context	Mathematical task
	Two sisters shared a description of vacuuming the house while their mother timed them.	Nirvana and Nora show aroha at home by helping with the chores. Here is a set of data that shows how many minutes tamariki spend doing chores on the weekend. Minutes: 0, 5, 23, 7, 18, 6, 23, 31, 7, 15, 23, 25, 60, 30, 95, 8, 19, 18, 28, 24, 10, 13, 55, 12, 5 Put these data into a stem and leaf graph. What does the graph show in relation to how many minutes tamariki spend doing chores on the weekend? Write statements.
	A child shared a photograph and described this as a Fijian masi made from bark of a tree that they had at home.	This is a Fijian masi. The brown dye is made from the bark of the mangrove tree and soot (ash from the fire) is used to make the black patterns. You have four and a half tubs of brown dye and it takes five-eighths of a tub to decorate each section. How many sections can you decorate before you need to make more dye?
	Two siblings shared a photo of the ‘ei katu that their Uncle made to sell.	Tiare and her Uncle are making an ‘ei katu with leaves and flowers. They make this pattern: Write the position number under pattern two. What would be in position 10? What would be in position 16? What would be in position 41? Explain how you know.

These group-worthy contextual mathematical tasks provide an illustration of how mathematical funds of knowledge can be built upon in the classroom. In the final interviews, the teachers reflected on their own learning across the length of the project. A common theme from the teachers was that being involved in the project had made them reflect upon their assumptions and also to consider how to more fully connect with students: “I’ve learnt to involve the tamariki more, so using their knowledge more, life experiences, probably not just in maths either.” For some teachers, this also included widening their own perspectives of mathematics: “Being able to see mathematics more across the board, and not just what I was taught from a textbook … It was the kids, it was their photos, they see it in really creative and different ways.” These opportunities for teachers to learn both about Pacific learners’ funds of knowledge related to mathematics and to develop group-worthy contextual tasks required both space away from teaching and time to undertake detailed planning sessions.

Key finding 4:
It is challenging for teachers to incorporate multiple knowledge bases of students throughout the enactment and use of the mathematical task during mathematics lessons; however, the use of reflective activities and purposeful planning supports this.

As shown in the previous section, the research team and teachers were able to successfully collaborate to plan mathematical tasks that were linked to multiple knowledge bases of diverse learners. Multiple knowledge bases include home, community, and cultural funds of knowledge and mathematical concepts and practices. A key challenge and learning for the teachers was how to incorporate multiple knowledge bases throughout the enactment and use of tasks during the lesson. Initially, teachers unevenly drew on the multiple knowledge bases of students in lessons, often predominantly aligning pedagogical practices with connections to mathematical concepts while largely ignoring the connections to home and cultural funds of knowledge. Video reflection and the use of purposefully designed reflective prompts and discussion were helpful in positioning the teachers to consider how to better connect across multiple knowledge bases. For example, one teacher stated:

I have been reflecting on my launch, we’ve come into lockdown now and on Zoom I had a couple of other students show me their axes at home but I didn’t really talk about that when we launched it. I just said ‘Oh there is a Samoan axe’, you know just ‘Who’s seen this before’ and kind of rushed into it. I should have asked if there were any other cultures that had this sort of axe and what it was used for but I didn’t really make those links through.

Other teachers shared similar reflections and discussed how they had realised that they had largely ignored the contextual features of the task and used the context simply to deliver the mathematical focus. In contrast, another teacher with a prompt from one of the research team had used the context to set up the norms of how to work collaboratively in mathematics:

We started talking about the axe and how, in order to make it, you have to work together and challenge yourself because it is hard to do and we do that in maths as well. Then we talked about how it is not usually just made by one person, you work together with your ‘aiga to do it together so it was a cool immediate connection between the norms and how to make the axe.

This led to a discussion of the cultural purposes of the axe and the cultural meanings of triangles which were the focus in the lesson:

Usually there are different types of ways to use the axe. I know one is for war and one is what the tau’olunga, what they use for dancing. I could have connected that and how it was made, by wood and everything, they worked together to find the proper wood.

Interestingly, some of the teachers were Pacific themselves with connections to Samoa and Tonga; however, they acknowledged that they had not considered the importance of introducing the cultural meaning of artefacts or activities when using contextual tasks in mathematics:

I guess that was my fault too with the planning, like even looking at the triangles, because triangles are really important, not only to Māori but [also] Pacifica culture. Triangles for Samoans are like shark teeth, which means protection, guidance sort of thing. You know, I never touch on any of that, but by just reflecting on it afterwards, I think ah man I could have, I could have brought that stuff in.

For both the Pacific teachers and non-Pacific teachers, this was an important learning experience in relation to honouring Pacific mathematical funds of knowledge. This focus continued through the project, illustrated by a teacher in her mid-project reflection who highlighted her learning as “the importance of the launch of the task to connect to the context for the children and how valuable it is to thoroughly think through all possible answers/thinking [mathematically] before teaching”. Similarly, at the end of the project in the final interviews, the teachers expressed both the importance of connecting to the context during the launch and throughout the lesson and also anticipating possible student responses in relation to mathematics: “When we do it, we talk about it, we think about what the anticipations might be and then we might go, ‘no it’s not good enough’. So there has just been a whole lot of conversation that has happened … we’re getting more in-depth anticipations.” We argue that opportunities for in-depth planning and reflection on practice are important components to develop pedagogical practices that draw on multiple knowledge bases of learners.

Key finding 5:
Use and enactment of group-worthy mathematical tasks that connect to funds of knowledge and cultural resources offer opportunities for students to both develop and strengthen their cultural identity and their mathematical learning.

A key aspect in this study was both the research team and teachers recognising both the funds of knowledge of Pacific learners and families and the cultural resources (language and values) that could be used as strengths in the classroom. Across the project, the teachers demonstrated their growing understanding of students as people within their family, community, and wider cultural group who enter schooling with funds of knowledge and existing mathematical understandings. From the classroom observations, it was evident that group-worthy mathematical tasks offered opportunities for the teachers to support students’ collective mathematical learning while also connecting to student funds of knowledge. Both interview data from the students themselves and teachers confirmed the potential of an approach of developing and enacting tasks based on funds of knowledge and cultural resources to both support mathematical learning, positive dispositions, and the development and maintenance of a strong cultural identity.

In the first example in this section, we use a classroom observation from the first year of the project on a Year 1 lesson to demonstrate how the teacher supported the development of mathematical learning using a group-worthy task linked to a familiar everyday context for the young students. One of the students from the class had shared a photograph (see Figure 7) and story which described how they were playing and sorting washing pegs.

The teachers used this as the basis of a task (see Figure 8) to introduce Year 1 students to repeating patterns. This was an introductory lesson with a focus on supporting the students to identify the unit of repeat in the pattern, copy and continue the pattern, and find further terms of the pattern.

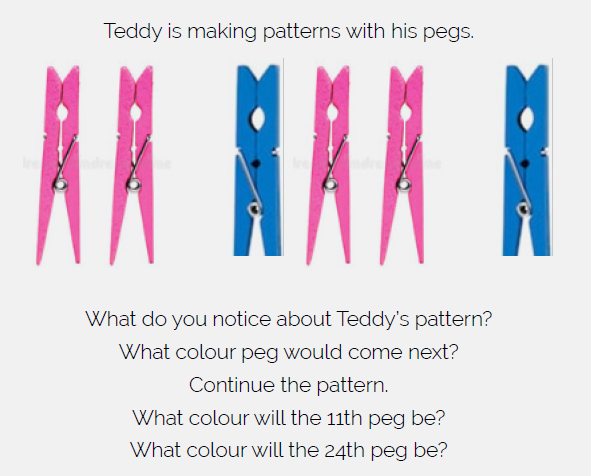
The teacher began by asking the students to describe the pattern that Teddy had made with the pegs. The students quickly verbally described the pattern in relation to the colours as: “pink, pink, blue, pink, pink, blue”. After the teacher prompted the students to discuss what they noticed, some of the students also connected to the pattern structure of the repeating pattern stating: “it goes two and a one, and then two and a one”. The teacher revoiced this and then asked other students to repeat to ensure that there was a collective focus on the structure of the pattern. In the next section of the lesson, the teacher asked the students to work collaboratively in pairs to continue the pattern. Following the paired work, the teacher asked the students to share their reasoning:

Teacher: What colour pegs would go next?

Student One: So it goes pink, pink, blue, pink, pink, blue [pointing to pattern in the task] and then another pink and then another pink [pointing to the spaces beyond the end of the pattern].

Teacher: Why did you think that?

Student Two: Because if you see there was another pink, pink here that would be the same.

Teacher: Oh, so you notice that it goes pink, pink, blue so it’s the same. Is that right?

Student One: Yeah, umm, so it would be a pink, a pink, and a blue.

Teacher: So a pattern is something that is the same.

Following these interactions in which the teacher worked with the students to establish the unit of repeat in the pattern and to ensure that they could copy and continue the pattern, the focus of the lesson then moved to identifying further terms. The teacher began by pointing at each peg in the pattern and showing the students how these could be associated with the terms of the pattern. She then provided the students with pictures of the coloured pegs and asked them to work in pairs to find the 11th term. The students were able to work together to copy and continue the pattern by using the pictures of the pegs to model the pattern and successfully identified that the 11th peg would be pink. At this point, the teacher then asked the students to notate the numbers below to start associating the pattern with the numerical order. Evident in the lesson was that, collectively, the students were able to develop their conceptual understanding of repeating patterns including identifying, copying, and continuing the pattern to find further terms. Following the lesson, the teacher reflected that the context of the tasks in using pegs to create a pattern was easily accessible

for the students especially as they were all familiar with using pegs at home when helping their parents to hang washing. She identified the student learning in relation to developing their understanding of what a pattern is and recognising that patterns are part of mathematics. In the second example using a classroom observation from a Years 5 and 6 lesson in the second year of the project, we illustrate how the teacher both connected to the student funds of knowledge and cultural values while also supporting students’ conceptual mathematical learning throughout the lesson. This lesson used a task shown in Table 2 which drew on a photograph that a student shared of Fijian masi (see Figure 9).

FIGURE 9. Task related to Fijian masi

This is a Fijian masi. The brown dye is made from the bark of the mangrove tree and soot (ash from the fire) is used to make the black patterns. You have four and a half tubs of brown dye and it takes five-eighths of a tub to decorate each section. How many sections can you decorate before you need to make more dye?

The teacher began the lesson by showing the picture of masi and supporting the students to share their own knowledge of masi. This led to students making connections to the varying names for masi across Pacific nations—siapo in Samoa and tapa in Tonga—and sharing their experiences and knowledge of this cultural artefact. This included the type of motifs and decorations used on masi, what it was used for in the home and community, and family connections in making and gifting masi. The teacher then highlighted the important cultural knowledge in relation to the use of natural resources to make something beautiful like masi and passing of knowledge: “It is a really old tradition and learned a long, long time ago and then been passed down through the generations.” In this way, the teacher both honoured Pacific knowledge and positioned student and family funds of knowledge as important. The teacher then connected to the process of making masi as an analogy of her expectation for how students would work together during the mathematics lesson: “When people make masi, traditionally they wouldn’t make it by themselves.” She then continued to describe the different roles that people would take to make masi (collecting materials from the mangroves and ash and through the process of creating bark-cloth) and used this to connect to how the students would take different roles while working in a group. This shows how teachers can both connect to student funds of knowledge and ways of being including cultural values during mathematics lessons.

To launch the task, the teacher first asked the students to imagine both fractional amounts (4 and ) and then to share their mathematical knowledge and understanding of these fractions. This led to students sharing their mathematical knowledge bases including the idea that representing of a tub involves splitting into eight parts because “the denominator is how many parts you have”. Following the launch, the students then worked in collaborative groups to solve the task. In the group recorded for the classroom observation, they began by representing the fractions in the task by drawing bar models of both fractional amounts. However, at this point, they had represented 4 as halves and were unsure how to continue to solve the task. As the teacher moved around the classroom, she questioned the group about their representation and helped them connect this to the context of the task: “So there is your four and a half tubs.” She then prompted them to consider how many eighths would be equivalent to one whole tub and how they could change the halves to eighths instead. In this way, the teacher connected to students’ mathematical understanding of fractions while also supporting them to maintain a link to the context of the task and use representations to solve the problem. The students were then able to solve the task by developing a solution strategy of converting the 4 into and iteratively adding for each section of the masi until they reached . This example illustrates how students collectively developed conceptual mathematical learning while the teacher also connected to their multiple knowledge bases including cultural funds of knowledge, cultural values including collectivism, mathematical concepts, and practices.

In the final interviews, students were asked to reflect on their favourite mathematics problems to solve over the year. Many students recalled specific tasks that were connected to their own funds of knowledge or shared funds of knowledge. This included both problems that connected to their culture, including artefacts and cultural activities, but also those that connected to household activities: “My one was the tiling one, because we actually did it in class and I liked that one because I was measuring.” Another student with Cook Island heritage shared her favourite tasks as those that involved ‘ei pupu and ‘ei katu: “It is part of my culture and it is very special to me because it is from the Cook Islands.” Students also reflected on their key learning in relation to their culture and mathematics: “When she talks about my culture, I feel happy because I didn’t know Samoan [culture] has maths in it” or “I feel really good because it is part of my culture and I want to teach other people what my culture is.” In this way, both the action of documenting their funds of knowledge and the teachers’ use of contextualised tasks positioned the students to see mathematics in their cultural identity.

Similarly, in the final interview, the teachers also made statements that reflected how they had noticed shifts in the students’ engagement, learning, and cultural identity. A common theme from the teachers was that using the tasks aligned with the cultural heritage of the students was both engaging and mana enhancing: “The look on kids’ faces when they know it is their photo or a friend from another class or ‘Oh I’ve got one of those in my house’. I guess that’s just good for their mana to see them.” Another teacher reflected on a Tongan female student she had in her class who was well below in her academic achievement: “We had a task around the taʻovala and just you know just the way she came out of her shell. You know she was able to explain and was so confident. It was like a natural thing she was able to just, she was the expert.” In these reflections, we see how the use of funds of knowledge based on the students’ experiences outside of school had both positive influence on their disposition and their mathematical learning.

Implications and recommendations for practice

1. Use of contextual mathematical tasks based on funds of knowledge and out-of-school experiences can have a positive impact on students’ mathematical learning, engagement, and disposition.
2. All learners from every cultural background have mathematical funds of knowledge and cultural resources that can be used as strengths in mathematics classrooms and beyond. Innovative methods such as photography and storytelling are useful ways to position children and families to document these funds of knowledge and share them with educators.
3. A key role for teachers and teacher educators is to be able to recognise the strands of mathematics evident in both cultural and everyday activities and artefacts. Teachers need to be learners of student, family, and community funds of knowledge. This requires teachers to recognise the expertise of families and children and invite them to share their out-of-school experiences that could be connected to mathematics.
4. Enacting tasks that draw on cultural, home, or community contexts requires care and thoughtful preparation to honour the context. Preparation regarding the context includes teachers drawing on a wide range of expertise (colleagues, teacher aides, families, and community members) to learn about cultural experiences or artefacts and then planning how to make genuine and authentic connections to the context throughout the mathematics lesson.
5. In-depth planning and preparation are needed to successfully draw on students’ multiple knowledge bases including their mathematical knowledge and understanding. This requires teachers to engage in considering the big mathematical ideas that underpin a task, map the multiple levels of mathematical learning, and anticipate student solution strategies and potential misconceptions.
6. During the launch of a contextual group-worthy task, teachers need to connect to the context of the task, position students as experts and assign them with status, and facilitate other students from differing cultural backgrounds to share their related funds of knowledge.
7. Teachers should be provided with opportunities to collaborate to develop tasks and to engage in reflection on their enactment of tasks and use of pedagogical practice during lessons. Specifically, videoreflection is an important tool coupled with the use of purposefully designed reflective prompts.

Conclusion

New Zealand, similar to many other Western countries, has had an inequitable schooling system in relation to mathematics teaching and learning for students from diverse cultural backgrounds (Turner et al., 2015; Young-Loveridge, 2009). For too long, Pacific student voices have been silenced within traditional mathematics classrooms by pedagogical practices and task design that only draws on the experiences and resources of the dominant cultural groups (Hunter & Hunter, 2018). This project aimed to position young Pacific learners and their families to document their mathematical funds of knowledge and share these with educators to support the develop of equity-focused mathematics teaching. We demonstrated that young Pacific learners and their families have rich funds of knowledge related to mathematics that is represented both in activities and artefacts. In this project, the crossing of boundaries between homes and schools provided possibilities for developing true power-sharing relationships. In collaboration with the research team, the teachers were able to plan group-worthy mathematical tasks that drew on students’ funds of knowledge. When these were enacted successfully in the classroom, this both enhanced students’ mathematical learning and disposition and provided an implicit message to students that they had the right to bring their funds of knowledge into the classroom and that these would be valued. We share these findings as an exemplar of how equitable mathematics classrooms can be developed.

Reference list

Aguirre J. M., Turner E. E., Bartell, T. G., Kalinec-Craig, C., Foote, M. Q., McDuffie, A. R., & Drake, C. (2013). Making connections in practice: How prospective elementary teachers connect to children’s mathematical thinking and community funds of knowledge in mathematics instruction. Journal of Teacher Education, 64(2), 178–192.

Averill, R., & Clark, M. (2012). Respect in teaching and learning mathematics. Set: Research Information for Teachers, 3, 50–57.

Bang, M., & Vossoughi, S. (2016). Participatory design research and educational justice: Studying learning and relations within social change making. Cognition and Instruction, 34(3), 173–193.

Bartell, T., Wager, A., Edwards, A., Battey, D., Foote, M., & Spencer, J. (2017). Toward a framework for research linking equitable teaching with the standards for mathematical practice. Journal for Research in Mathematics Education, 48(1), 7–21.

Betts, P., & Rosenberg, S. (2016). Making sense of problem solving and productive struggle. Delta-K, 53(2), 26–31.

Civil, M. (2016). STEM learning research through a funds of knowledge lens. Cultural Studies of Science Education, 11(1), 41–59.

Clarke, D., & Roche, A. (2017). Using contextualized tasks to engage students in meaningful and worthwhile mathematics learning. Journal of Mathematical Behaviour, 51, 95.

Cumbo, B., & Selwyn, N. (2022). Using participatory design approaches in educational research. International Journal of Research & Method in Education, 45(1), 60–72.

Esteban-Guitart, M. (2012). Towards a multi-methodological approach to identification of funds of identity, small stories and master narratives. Narrative Inquiry, 22, 173–180.

Hunter, J. (2022). Challenging and disrupting deficit discourses in mathematics education: Positioning young diverse learners to document and share their mathematical funds of knowledge. Research in Mathematics Education, 24(2), 187–201.

Hunter, J., Hunter, R., Tupounuia, J., & Fitzgerald, L. (2021). Implementing localised curriculum drawing on a funds of knowledge approach: Teacher perceptions and challenges. New Zealand Annual Review of Education, 26, 153–161.

Hunter, R., & Hunter, J. (2018). Maintaining a cultural identity while constructing a mathematical disposition as a Pāsifika learner. In E. A. McKinley & L. Tuhiwai Smith (Eds), Handbook of indigenous education (pp. 1–19). Springer.

Joves, P., Siques C., & Esteban-Guitart, M. (2015). The incorporation of funds of knowledge and funds of identity of students and their families into educational practice. A case study from Catalonia, Spain. Teaching and Teacher Education, 49, 68–77.

Kelly, A. (2013). Research as design. Educational Researcher, 32(1), 3–4.

Kelly, A. E., Lesh, R. A., & Baek, J. Y. (2014). Handbook of design research methods in education: Innovations in science, technology, engineering, and mathematics learning and teaching. Routledge.

Langer-Osuna, J. (2016). The social construction of authority among peers and its implications for collaborative mathematics problem solving. Mathematical Thinking & Learning, 18(2), 107–124.

Liebenberg, L. (2018). Thinking critically about photovoice: Achieving empowerment and social change. International Journal of Qualitative Methods, 17(1).

Llopart, M., & Esteban-Guitart, M. (2017). Strategies and resources for contextualising the curriculum based on the funds of knowledge approach: A literature review. Australian Educational Researcher, 44, 255–274.

Mills, K., Bonsignore, E., Cleggetal, T., Ahn, J., Yip, J., Pauw, D., Cabrera, L., Hernly, K., & Pitt, C. (2019). Connecting children’s scientific funds of knowledge shared on social media to science concepts. International Journal of Child Computer Interaction, 21, 54–64.

Moje, E. B., Ciechanowski, K. M., Kramer, K., Ellis, L., Carrillo, R., & Collazo, T. (2004). Working toward third space in content area literacy: An examination of everyday funds of knowledge and discourse. Reading Research Quarterly, 39(1), 38–70.

Moll, L. C., Amanti, C., Neff, D., & Gonzalez, N. (1992). Funds of knowledge for teaching: Using a qualitative approach to connect homes and classrooms. Theory into Practice, 31, 132–141.

National Council of Teachers of Mathematics. (2014). Principles to actions: Ensuring mathematical success for all. Author.

Parker, F., Bartell, T., & Novak, J. (2017). Developing culturally responsive mathematics teachers: Secondary teachers’ evolving conceptions of knowing students. Journal of Mathematics Teacher Education, 20, 385–407.

Rubel, L. H., & McCloskey, A. V. (2021). Contextualization of mathematics: Which and whose world? Educational Studies in Mathematics, 107(2), 383–404.

Smith, M. S., & Stein, M. K. (2011). Five practices for orchestrating productive mathematics discussion. National Council of Teachers of Mathematics.

Sutton-Brown, C. A. (2014). Photovoice: A methodological guide. Photography and Culture, 7(2), 169–185.

Takeuchi, M. A. (2018). Conversions for life: Transnational families’ mathematical funds of knowledge. In T. G. Bartell (Ed.), Toward equity and social justice in mathematics education (pp. 127–143). Springer.

Trinick, T., & Heaton, S. (2020). Curriculum for minority Indigenous communities: Social justice challenges. Language, Culture and Curriculum, 34(3), 273–287. https://doi.org/10.1080/07908318.2020.1831009

Turner, E. E., Drake, C., McDuffie, A. R., Aguirre, J., Bartell, T. G., & Foote, M. Q. (2012). Promoting equity in mathematics teacher preparation: A framework for advancing teacher learning of children’s multiple mathematics knowledge bases. Journal of Mathematics Teacher Education, 15, 67–82.

Turner, H., Rubie-Davies, C. M., & Webber, M. (2015). Teacher expectations, ethnicity and the achievement gap. New Zealand Journal of Educational Studies, 50, 55–69.

Wernet, J. W. (2017). Classroom interactions around problem contexts and task authenticity in middle school mathematics. Mathematical Thinking and Learning: An International Journal, 19(2), 69–94.

Williams, J. J., Tunks, J., Gonzalez-Carriedo, R., Faulkenberry, E., & Middlemiss, W. (2020). Supporting mathematics understanding through funds of knowledge. Urban Education, 55(3), 476–502.

Young-Loveridge, J. (2009). Patterns of performance and progress of NDP students in 2008. Findings for the New Zealand numeracy development project 2008 (pp. 12–26). Ministry of Education.

Zipin, L., Sellar, S., & Hattam, R. (2012) Countering and exceeding ‘capital’: A ‘funds of knowledge’ approach to re-imagining community. Discourse: Studies in the Cultural Politics of Education, 33(2), 179–192.

Project team

Professor Jodie Hunter is a Professor in Mathematics Education in the Institute of Education at Massey University. Jodie’s research is focused on developing equitable outcomes in mathematics education for diverse groups of students.

Email: j.hunter1@massey.ac.nz

Emeritus Professor Roberta (Bobbie) Hunter was a Professor in Pasifika Education in the Institute of Education at Massey University. Bobbie’s research focuses on culturally responsive teaching for Pacific students and how teachers develop mathematical practices in classrooms.

Dr John Tupouniua is a Lecturer in Mathematics Education in the Institute of Education at Massey University. John’s research interests include the development of algorithmic thinking and making mathematics accessible to all learners.

Louise Fitzgerald is a Lecturer in Mathematics Education and a PhD student at the Institute of Education at Massey University. Louise’s research interests include teaching issues of social justice through primary school mathematics.